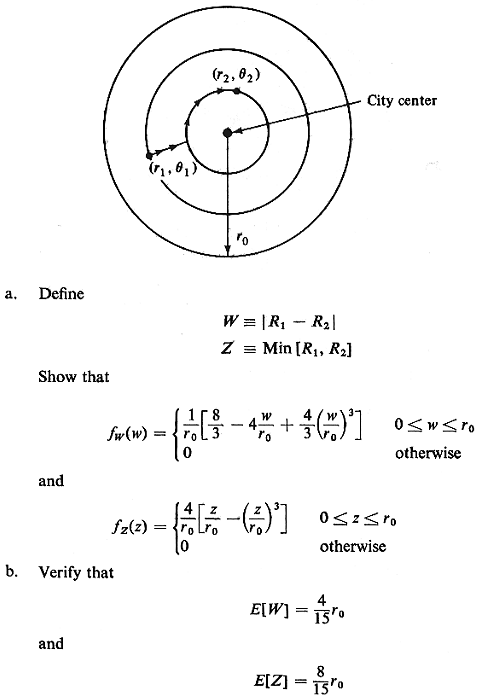
3.27 Circular city,
revisited Suppose
that two points (R1, 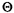 2)
and (R2, 2)
and (R2, 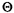 2) are
independently, uniformly distributed over a circular city of radius
ro
and area A= 2) are
independently, uniformly distributed over a circular city of radius
ro
and area A= 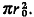 Suppose further that this city has a
large number of radial
routes and circular ring routes so that the travel distance between
(R1, Suppose further that this city has a
large number of radial
routes and circular ring routes so that the travel distance between
(R1,
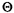 1)
and (R2, 1)
and (R2, 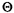 2) can be
accurately approximated as 2) can be
accurately approximated as
D = |R1 - R2| + Min [ R1, R2] |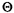 1
- 1
- 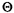 2| 2|
where 0  | |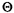 1 - 1 - 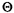 2| 2|
  signifies
the magnitude of the angular difference between signifies
the magnitude of the angular difference between 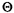 1
and 1
and 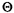 2. In words,
travel from an outer point, say,
(R1, 2. In words,
travel from an outer point, say,
(R1,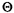 1)
if R, > R2, to an inner point
(R2, 1)
if R, > R2, to an inner point
(R2,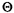 1)
first occurs along a radial route to a ring located a distance R2
from the city center,
and then along that ring (in the direction of minimum travel
distance) to (R2, 1)
first occurs along a radial route to a ring located a distance R2
from the city center,
and then along that ring (in the direction of minimum travel
distance) to (R2,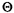 1);
the same path is traveled in reverse if travel is from
(R2, 1);
the same path is traveled in reverse if travel is from
(R2,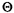 1)
to (R1, 1)
to (R1,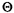 1).
A sample path is shown in Figure P3.27. 1).
A sample path is shown in Figure P3.27.
and thus
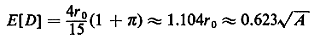
Compare this result to analogous results in
Problems 3.20 and 3.21.
|