


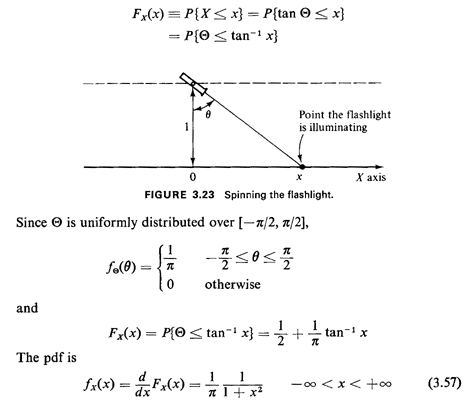
3.3.3 Cauchy DistributionAs illustrated above, many geometrically oriented problems require deriving the distribution of a function of one or more random variables. Often the original random variable(s) is (are) uniformly, independently distributed over some range of values, perhaps depicting position or angle of an object. One simple example gives rise to an unusual probability law, the Cauchy distribution.Suppose that Buffon's needle were, in fact, a narrow-beam flashlight rather than a needle. The flashlight is spun around its center, located unit distance from the x-axis (Figure 3.23). After the flashlight has stopped spinning, we are interested in the point X on the x-axis at which the flashlight is pointing. If it is not pointing toward the x-axis, we repeat the spinning trial as many times as necessary until it does. This situation might, in fact, correspond to a method for choosing (1) a point for random inspection along a pipeline, (2) a volunteer standing in a line of personnel at "rollcall," or (3) a "winner" in a "straight" version of spin- the-bottle. As shown in Figure 3.23 the point X is determined by the angle
This is the Cauchy probability density function. It possesses the interesting property that its variance is infinite (since the integral 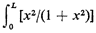 tends to L as L becomes large). In fact, to be mathematically
precise, the mean of the Cauchy density function is
also undefined since the integral
tends to L as L becomes large). In fact, to be mathematically
precise, the mean of the Cauchy density function is
also undefined since the integral
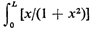 dx tends to
dx tends to  n L as L becomes large. Thus, a
perfectly valid
random variable need not possess well-defined or finite means and
variances. n L as L becomes large. Thus, a
perfectly valid
random variable need not possess well-defined or finite means and
variances.
Problem 3.17 asks you to explore certain additional properties of the Cauchy distribution. |


