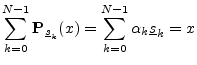Theorem: The projections of any vector
We now arrive finally at the main desired result for this section:
Theorem: The projections of any vector
![]() onto any orthogonal basis set
for
onto any orthogonal basis set
for ![]() can be summed to reconstruct
can be summed to reconstruct ![]() exactly.
exactly.
Proof: Let
![]() denote any orthogonal basis set for
denote any orthogonal basis set for ![]() .
Then since
.
Then since ![]() is in the space spanned by these vectors, we have
is in the space spanned by these vectors, we have
![$\displaystyle {\bf P}_{\sv_k}(\sv_l) \isdef
\frac{\left<\sv_l,\sv_k\right>}{\l...
...ay}{ll}
\underline{0}, & l\neq k \\ [5pt]
\sv_k, & l=k. \\
\end{array}\right.
$](img969.png)