Graphical Computation of
Amplitude Response from
Poles and Zeros

Now consider what happens when we take the factored form of the general transfer function, Eq. (8.2), and set $z$ to $e^{j\omega T}$ to get the frequency response in factored form:

$$H(e^{j\omega T}) = g\frac{(1-q_1e^{-j\omega T})(1-q_2e^{-j\omega ... ...ga T})}{(1-p_1e^{-j\omega T})(1-p_2e^{-j\omega T})\cdots(1-p_Ne^{-j\omega T})}$$

As usual for the frequency response, we prefer the polar form for this expression. Consider first the amplitude response $G(\omega) \isdef \left\vert H(e^{j\omega T})\right\vert$.

$$G(\omega) = \left\vert g\right\vert\frac{\left\vert 1-q_1e^{-j\omega T}\right... ... 1-p_2e^{-j\omega T}\right\vert\cdots\left\vert 1-p_Ne^{-j\omega T}\right\vert}$$

$$= \left\vert g\right\vert \frac{\left\vert e^{-jM\omega T}\right\ve... ...vert e^{j\omega T}-p_2\right\vert\cdots\left\vert e^{j\omega T}-p_N\right\vert}$$

$$= \left\vert g\right\vert \frac{\left\vert e^{j\omega T}-q_1\right\... ...\omega T}-p_2\right\vert\cdots\left\vert e^{j\omega T}-p_N\right\vert} \protect$$ (9.3)

Figure 8.1: Treatment of complex numbers as vectors in a plane.

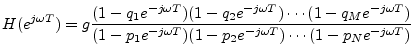
In the complex plane, the number $z = x + jy$ is plotted at the coordinates $(x, y)$ [83]. The difference of two vectors $u = x_1 + jy_1$ and $v = x_2 + jy_2$ is $u - v = (x_1 - x_2) + j(y_1 - y_2)$, as shown in Fig.8.1. Translating the origin of the vector $u-v$ to the tip of $v$ shows that $u-v$ is an arrow drawn from the tip of $v$ to the tip of $u$. The length of a vector is unaffected by translation away from the origin. However, the angle of a translated vector must be measured relative to a translated copy of the real axis. Thus the term $e^{j\omega T} - q_i$ may be drawn as an arrow from the $i$th zero to the point $e^{j\omega T}$ on the unit circle, and $e^{j\omega T} - p_i$ is an arrow from the $i$th pole. Therefore, each term in Eq. (8.3) is the length of a vector drawn from a pole or zero to a single point on the unit circle, as shown in Fig.8.2 for two poles and two zeros.

$\parbox{0.8\textwidth}{% The frequency response magnitude (amplitude... ... by the product of lengths of vectors drawn from the poles to $e^{j\omega T}$.}$

Figure 8.2: Measurement of amplitude response from a pole-zero diagram. A pole is represented in the complex plane by 'X'; a zero, by 'O'.

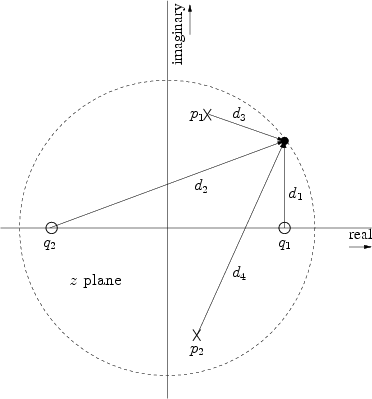For example, the dc gain is obtained by multiplying the lengths of the lines drawn from all poles and zeros to the point $z = 1$. The filter gain at half the sampling rate is the product of the lengths of these lines when drawn to the point $z = -1$. For an arbitrary frequency $f$ Hz, we draw arrows from the poles and zeros to the point $z = e^{j2\pi fT}$. Thus, at the frequency where the arrows in Fig.8.2 join, (which is slightly less than one-eighth the sampling rate) the gain of this two-pole two-zero filter is $G(\omega) = (d_1d_2)/(d_3d_4)$. 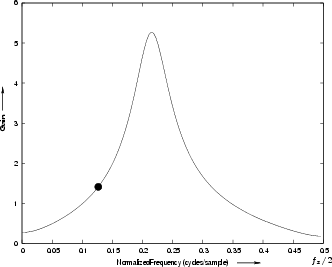Figure 8.3 gives the complete amplitude response for the poles and zeros shown in Fig.8.2. Before looking at that, it is a good exercise to try sketching it by inspection of the pole-zero diagram. It is usually easy to sketch a qualitatively accurate amplitude-response directly from the poles and zeros (to within a scale factor).

Figure 8.3: Amplitude response obtained by traversing the entire upper semicircle in Fig.8.2 with the point $e^{j\omega T}$. The point of the amplitude obtained in that figure is marked by a heavy dot. For real filters, precisely the same curve is obtained if the lower half of the unit circle is traversed, since $G(\omega ) = G( - \omega )$. Thus, plotting the response over positive frequencies only is sufficient for real filters.